Ionic compounds are different from those containing only covalent bonds. The latter exist as molecules whether in the pure state (crystals, liquids) or in solution. The former are a combination of two different kinds of particles. One kind (cations) carry a positive charge, and the second kind (anions) carry a negative charge. Sodium chloride is a typical ionic compound. It exists not as sodium chloride units but as sodium ions and chloride ions. In the solid state, these ions are arranged in a three-dimensional lattice, one kind of ion alternating with the other. In solution, the sodium ions and the chloride ions behave independently of each other.

Consider a particular example of this independent behavior. Suppose you have two solutions. One contains silver ions and an anion whose silver salt is soluble. The second contains chloride ions and a cation whose chloride is soluble. When the two solutions are combined, a white precipitate of silver chloride forms. We can write an equation for the reaction:

Ag⁺ + Cl^- AgCl ()

The other ions are still present in the solution surrounding the precipitate (the supernatant liquid), but they do not participate in the reaction (see Figure 11.5a).

Earlier we would have written the equation differently. Assuming that nitrate was the anion with silver and sodium the cation with chloride, the equation would have been

AgNO₃ + NaCl AgCl () + NaNO₃

Another way to write the equation shows the ionic compounds in solution as separate ions:

Ag⁺ + NO₃^- + Na⁺ + Cl^- AgCl()+ Na⁺ + NO₃^-

This last equation shows clearly that only the silver and the chloride ions take part in the reaction; the sodium and nitrate ions do nothing. These nonparticipating ions are called spectator ions, meaning that they do not participate in the reaction - just as people in the stands at a football game are spectators, meaning that they do not participate in the game. The best equation for a reaction is one that shows only the participating ions; such an equation is called the net ionic equation. The net ionic equation for the reaction of silver ion with chloride is

Ag⁺ + Cl^- AgCl ()

Consider another situation. When an acid reacts with a hydroxide, water and a salt are formed. All acids release hydrogen ions in solution. Hydroxides furnish hydroxide ions. Hydrogen ions and hydroxide ions react to form water, a covalent un-ionized molecule (see Figure 11.5b). The equation for this reaction is

H⁺ + OH^- H₂O

Previously we would have written this neutralization reaction using complete formulas. For example, the equation for the reaction of hydrochloric acid with sodium hydroxide would be

HCl + NaOH NaCl + H₂O

Rewriting this equation to show the acid, the hydroxide, and the salt as ions - which is the way these compounds exist in solution - would give the equation

H⁺ + Cl^- + Na⁺ + OH^- Na⁺ + Cl^- + H₂O

This equation shows clearly that the hydrogen and the hydroxide ions react and that the sodium and chloride ions are spectator ions. They would not appear in the net ionic equation.

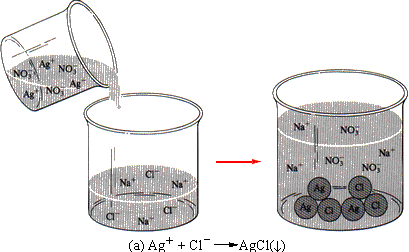
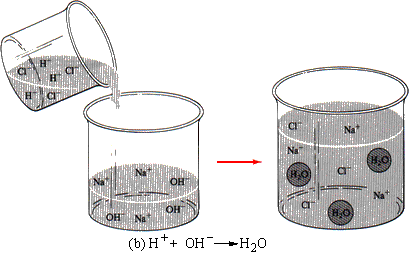
FIGURE 11.5 (a) When a solution of silver nitrate is added to a solution of sodium chloride, the silver ions combine with the chloride ions to form a precipitate of silver chloride. The sodium and the nitrate ions are nonparticipating spectator ions. (b) When hydrochloric acid is added to a solution of potassium nitrate, the hydrogen ions of the acid combine with the hydroxide ions of the potassium hydroxide to form molecules of water. The chloride and potassium ions are nonparticipating spectator ions.

Mg + 2 H⁺ Mg²⁺ + H₂

Again, the identity of the anion of the acid is not important. It is present as a spectator ion and need not be shown in the equation.

Like all equations, net ionic equations must be balanced. Previously we have been concerned only with balancing equations by numbers and kinds of atoms. Now we must also be certain that equations are balanced by charge; that is, the total charge on the reactants must equal the total charge on the products.

Example: a. Write the net ionic equation for the formation of the insoluble compound copper(II) sulfide. b. Write the net ionic equation for the formation of a precipitate of calcium phosphate. For each reaction name the appropriate spectator ions. Solution a. The formula of copper (II) sulfide is CuS. The cation is Cu2+; the anion is S2-. the net ionic equation for the formation of Cus would show only these two ions. Cu2+ + S2- CuS Whatever anion is with Cu2+ and whatever cation is with S2-; in the reactants do not take part in the reaction and are spectator ions. Appropriate spectator ions might be nitrate as anion and sodium as cation. We chose these ions because the reactants must be soluble and both nitrates and sodium salts are always soluble. b. The formula of calcium phosphate is Ca3(PO4)2. The cation is Ca2+; the anion is PO43-. The net ionic equation is 3 Ca2+ + 2 PO43- Ca3(PO4)2 Note that the net charge on the reactants is zero as is that on the product. Appropriate spectaor ions would be nitrate as anion, sodium as cation.

Net ionic equations can be derived from complete equations. The complete equation is written first. It is examined to determine whether there has been a change in oxidation numbers. If there has been such a change, those ions or molecules that contain the atoms changing oxidation number are isolated for the net ionic equation. If no oxidation numbers change, the products are examined to find whether a covalent compound was formed. If so, the ions that combined to form that molecule are isolated for the net ionic equation. If all the products are ionic, and no oxidation number change occurred, one of the products must be a precipitate. The solubility rules in Table 8.3 are used to determine which product is the precipitate, and the ions it contains are isolated for the net ionic equation.

Example: Write the net ionic equation for the following: a. the reaction between silver nitrate and potassium sulfate b. the reaction of sulfuric acid with sodium hydroxide c. the diplacement of bromine from sodium bromide by chlorine Solution a. The complete balanced equation for the reaction is: There have been no changes in oxidation numbers. Neither producct is covalent. According to the solubility rules, silver sulfate is insoluble. Isolating the ions it contains gives the net ionic equation This equation can be checked against the complete ionic equation in which we have crossed out the nonparticipating or spectator ions. b. The complete balanced equation for the reaction is the oxidation numbers are shown and none has changed. Water is covalent the other compounds are ionic. The net ionic equation for the reaction should be Writing the complete equation in ionic form gives: and crossing out the spectator ions shows that our choice for the net ionic equation was correct. c. The complete equation for the reaction is From the oxidation numbers, we see that both bromine and chloride have changed oxidation number. Isolating these ions gves the net ionic equation The complete ionic equation is Crossing out the spectator sodium ions gives the same net ionic equation.